Equation of continuity
An ideal fluid is incompressible. In this case, the equation of continuity holds true for it.
Equation of continuity
|
|
If the fluid flow is incompressible, the product of the cross-section area of the tube and the flow speed of the fluid is constant along the same tube. This constant, as we have seen, is the volumetric flow rate. \[A_1 \cdot v_1 = A_2 \cdot v_2 = \mathrm{const} = I\] This means that when the tube gets narrower, the fluid will speed up, and conversely, it will slow down in broader parts. |
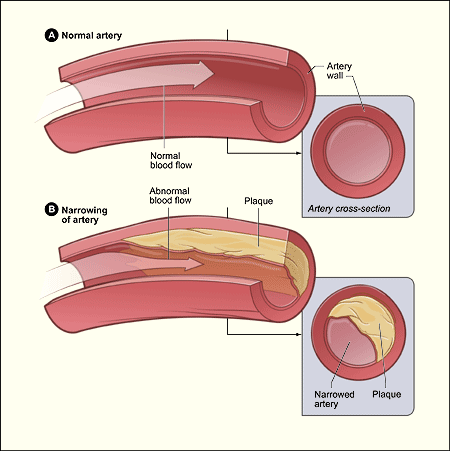
Arteriosclerosis
|
Arteriosclerosis is a condition in which the wall of the artery thickens, hardens and loses its elasticity. It usually means that the inner cross-section of the artery is reduced. If the inner diameter of the healthy artery wall is \(d_1 = 2.25\,\mathrm{cm}\) and the normal flow speed of blood here is \(v_1 = 40\,\frac{\mathrm{cm}}{\mathrm{s}},\) what will be the flow speed in the sclerotic section, where the diameter is reduced to \(d_2 = 1.5\,\mathrm{cm}\)? To answer the question, we can use the equation of continuity. We shall need the cross-section areas in the healthy part and in the narrowed part: \[A_1 = r_1^2 \cdot \pi = \left(\frac{d_1}{2}\right)^2 \cdot \pi,\] and \[A_2 = r_2^2 \cdot \pi = \left(\frac{d_2}{2}\right)^2 \cdot \pi.\] The equation of continuity: \[A_1 \cdot v_1 = A_2 \cdot v_2.\] Rearranging, we get \[v_2 = v_1 \cdot \frac{A_1}{A_2} = v_1 \cdot \frac{d_1 ^2 \cdot \pi / 4}{d_2^2 \cdot \pi / 4} = v_1 \cdot \frac{d_1^2}{d_2^2} = v_1 \cdot \left(\frac{d_1}{d_2}\right)^2 = 40\,\frac{\mathrm{cm}}{\mathrm{s}} \cdot \left(\frac{2.25\,\mathrm{cm}}{1.5\,\mathrm{cm}}\right)^2 = 40\,\frac{\mathrm{cm}}{\mathrm{s}} \cdot \left(1.5\right)^2 = 90\,\frac{\mathrm{cm}}{\mathrm{s}}.\] So the flow speed in the narrowed part is \(90\,\frac{\mathrm{cm}}{\mathrm{s}}.\) |
Proof for the equation of continuity
|
|
Consider an incompressible fluid flow as shown in the figure. The volume of the flow space between (1) and (2) is constant, and the condition of incompressibility means that since the density \(\varrho\) is constant, the mass of the fluid between (1) and (2) must also stay constant. This means that the mass flowing in in unit time at (1) must be equal to the mass flowing out in unit time at (2). Again using the condition that the flow is incompressible and thus the density is constant, this also means that the volume flowing in in unit time at (1) is equal to the volume flowing out in unit time at (2). But we have seen that the volume carried by the flow in unit time, that is, the volumetric flow rate, is equal to the product of the cross section and the flow speed: \[\Delta V_1 = I_1 \cdot \Delta t = \Delta V_2 = I_2 \cdot \Delta t,\] \[I_1 = I_2,\] \[I = A \cdot v,\] \[A_1 \cdot v_1 = A_2 \cdot v_2.\] Since cross section (1) and (2) were chosen arbitrarily, this relationship must hold for any two cross sections in the tube. |